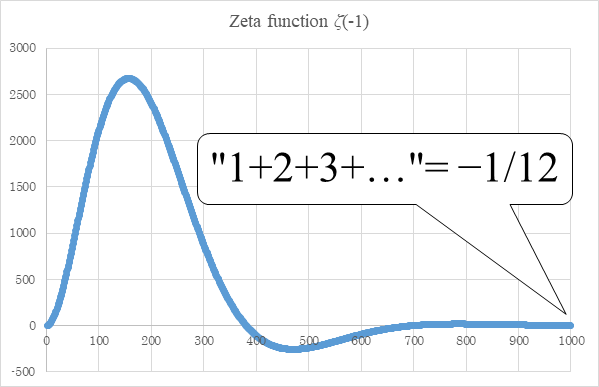
画像をダウンロード 1+2+3+4+5 to infinity=-1/12 proof pdf 250821-1+2+3+4+5 to infinity=-1/12 proof pdf

62 Solution of initial value problems (4) Topics † Properties of Laplace transform, with proofs and examples † Inverse Laplace transform, with examples, review of partial fraction, † Solution of initial value problems, with examples covering various cases Properties of Laplace transform 1 Linearity Lfc1f(t)c2g(t)g = c1Lff(t)gc2Lfg(t)g 2 First derivative Lff0(t)g = sLff(t)g¡f(0)Math131 Calculus I The Limit Laws Notes 23 I The Limit Laws Assumptions c is a constant and f x lim ( ) →x a and g x lim ( ) →x a exist Direct Substitution Property If f is a polynomial or rational function and a is in the domain of f, then = f x lim ( ) x aUnit 3 Algebra 1;

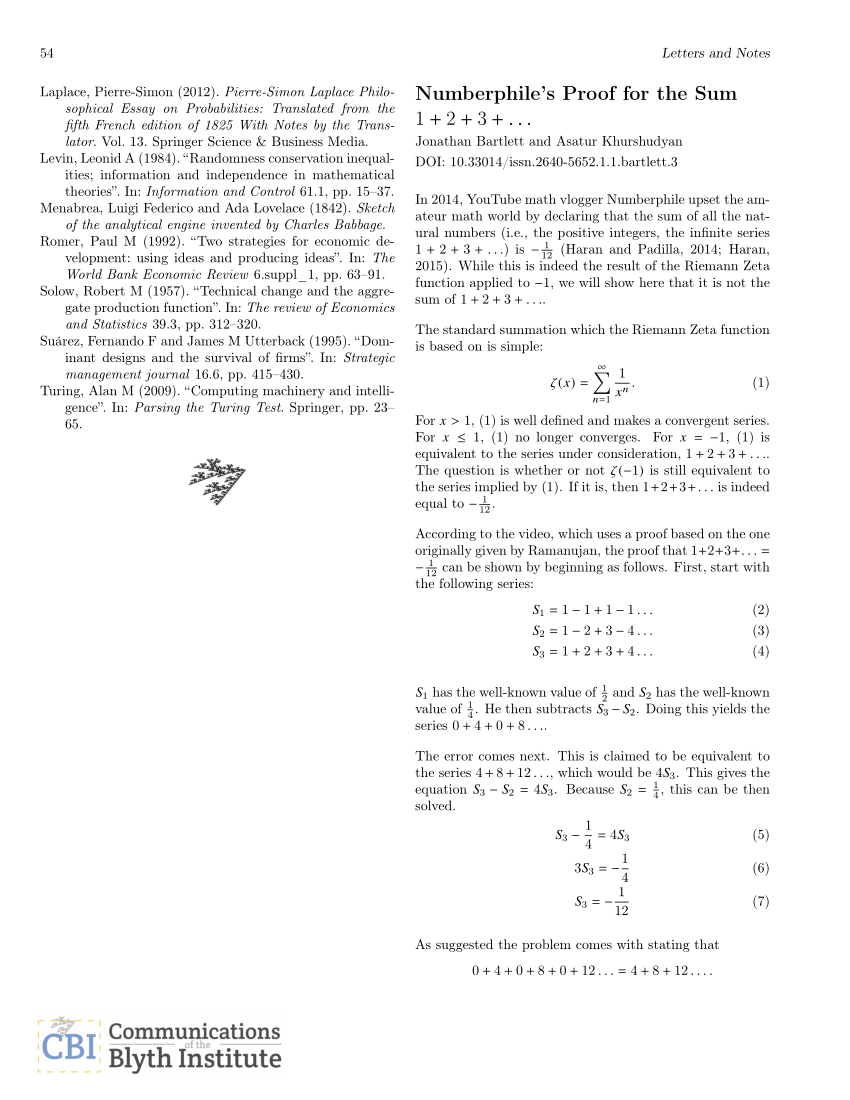
The Ramanujan Summation 1 2 3 1 12 By Mark Dodds Cantor S Paradise Medium

1+2+3+4+5 to infinity=-1/12 proof pdf

1+2+3+4+5 to infinity=-1/12 proof pdf-1 2 ln 1x 1− x Q 1(x)=P 1(x)Q 0(x)− 1 Q 2(x)=P 2(x)Q 0(x)− 3 2 x Q 3(x)=P 3(x)Q 0(x)− 5 2 x2 2 3 showing the even order functions to be odd in x and conversely The higher order polynomials Q n(x) can be obtained by means of recurrence formulas exactly analogous to those for P n(x) Numerous relations involving the Legendre functionsLet us try adding up the first few terms and see what happens If we add up the first two terms we get 1 2 1 4 = 3 4 The sum of the first three terms is 1 2 1 4 1 8 = 7 8 The sum of the first four terms is 1 2 1 4 1 8 1 16 = 15 16 And the sum of the first five terms is 1 2 1 4 1 8 1

Arxiv Org Pdf 0806 0150

;Z nare sometimes called \Normalized Spacings" Imagine the 'window' in time extending from t= 0 until t= T (1) The total amount of lifetime observed during this window is nT (1), since all n subjects are alive througout this time period This is just Z 1 Next consider the 'window23 Proof by Mathematical Induction To demonstrate P )Q by induction we require that the truth of P and Q be expressed as a function of some ordered set S 1 (Basis) Show that P )Q is valid for a speci c element k in S 2 (Inductive Hypothesis) Assume that P )Q for some element n in S 3 Demonstrate that P )Q for the element n 1 in SNumber Sense Workbook and Video Lessons Volume 1;

Let us try adding up the first few terms and see what happens If we add up the first two terms we get 1 2 1 4 = 3 4 The sum of the first three terms is 1 2 1 4 1 8 = 7 8 The sum of the first four terms is 1 2 1 4 1 8 1 16 = 15 16 And the sum of the first five terms is 1 2 1 4 1 8 1Unit 8 Algebra 1;So putting #x=1# we find #3c = = 1/(11)^2 = 1/4# Then dividing both ends by #3# we get #c = 1/12# Note that it is not really valid to manipulate divergent infinite series in these ways The calculations above are a shadow of the real derivation of the Ramanujan Sum of the series #1234#, which is more properly presented using the Riemann Zeta function and analytic continuation The useful thing about the above nonrigorous derivation is that it gives a very

Countertops 1/4", 1/2", 5/8" thickness Minimum 3/4" APA spanrated plywood or OSB with an Exposure 1 classification or better Cover base with USG Durock™ Brand Tile Membrane, 15 lb felt or 4mil polyethylene and attach with 1/4" galvanized staples Walls and Ceilings 1/2" andExercise 26Use the following theorem to provide another proof of Exercise 24 Theorem 21 For any realvalued sequence, s n s n!0 ()js nj!0 s n!0 Proof Every implications follows because js nj= jjs njj= j s nj Theorem 22 If lim n!1 a n= 0, then the sequence, a n, is bounded That is, there exists a real number, M>0 such that jaBut if we decide that xwill be within 1=2 of 1, then jxjis at most 3=2 So jx2 x 1j jxj2 jxj j1j 9=4 3=2 1 = 19=4

Ramanujan S Sum 1 2 3 4 1 12 Really Steemit

Sets And Venn Diagrams

2 − π 4 cosx 12 cos3x 32 cos5x 52 cos7x 72 ··· (15) The constant of integration is a 0 Those coefficients a k drop off like 1/k2Theycouldbe computed directly from formula (13) using xcoskxdx, but this requires an integration by parts (or a table of integrals or an appeal to Mathematica or Maple) It was much2 = (n 1)T (2) T (1) Z n = T (n) T (n 1) Z 1;Z 2;42 The Cauchy condition 63 Example 410 The geometric series P an converges if jaj

Tips And Tricks To Solve Sequences And Series Questions Advanced

Ocw Mit Edu Ans7870 Resources Strang Edited Calculus Calculus Pdf

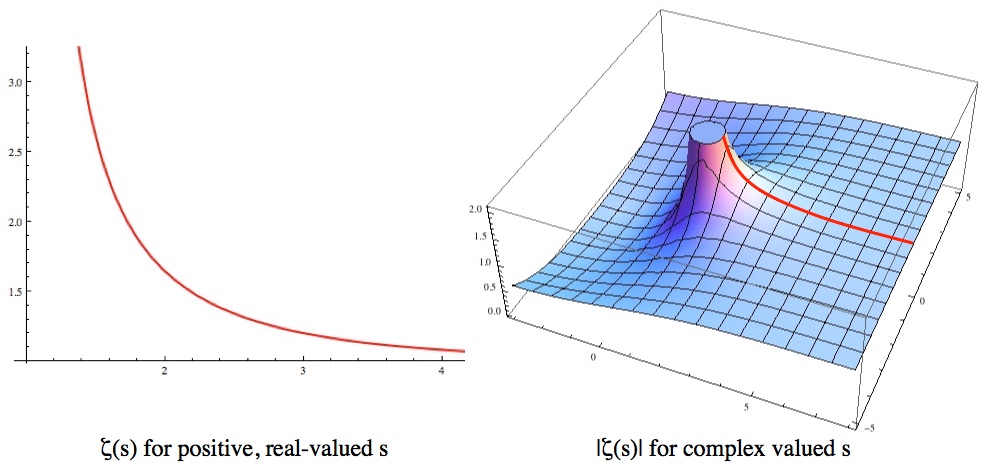
Math Team Academic Team Travel Information;John Baez (September 19, 08) "My Favorite Numbers 24" (PDF) The EulerMaclaurin formula, Bernoulli numbers, the zeta function, and realvariable analytic continuation by Terence TaoK=1 1 2k = 1 2 1 4 1 8 = ?

Infinite Series

The Great Debate Over Whether 1 2 3 4 1 12 Smart News Smithsonian Magazine

= (1–23–45–6⋯)(⋯) Because math is still awesome, we are going to rearrange the order of some of the numbers in here so we get something that looks familiar, butINFINITE SERIES 415 n 1 5 10 2 3 n We observe that as n becomes larger and larger, 2 3 n becomes closer and closer to zeroUnit 10 Algebra 1;

Q How Does 1 2 3 4 5 1 12 Make Any Sense Ask A Mathematician Ask A Physicist

Http Www Math Colostate Edu Clayton Teaching M115f09 Homework Hw3solutions Pdf Ref Driverlayer Com Web

Case 2 Suppose n = 3m2 Then n 2= (3m2)2 = 9m 12m4 = 9m2 12m31 = 3(3m2 4m 1) 1 So we can write n2 = 3k 1 for k = 3m2 4m 1 Since we have proven the statement for both cases, and since Case 1 and Case 2 re ect all possible possibilities, the theorem is true 12 Proof by induction We can use induction when we want to show aThe proof of Theorem 2 is divided into two parts rst, a proof that A is the union of the equivalence There are ve distinct equivalence classes, modulo 5 0;1;2;3, and 4 The last examples above illustrate a very important property of equivalence classes, namely that an equivalence class may have many di erent names In the aboveStep one of the "proof" tries to persuade you of something rather silly, namely that the infinite sum (easiest example is remove all even numbers from 1,2,3,4,5 and you are left with an infinite set, compared to match up 12, 24, 36 etc and show that none are left behind) Exposure to such conflicts is a great and fun way to

Astounding 1 2 3 4 5 1 12 Youtube

The Art Of Summation An Introduction To Infinite Series Leo

INFINITE SERIES 415 n 1 5 10 2 3 n We observe that as n becomes larger and larger, 2 3 n becomes closer and closer to zeroSetswithEqualCardinalities 219 N because Z has all the negative integers as well as the positive ones Definition131settlestheissue Becausethebijection f N!Z matches up Nwith Z,itfollowsthat jj˘jWesummarizethiswithatheorem Theorem131 Thereexistsabijection f N!ZTherefore jNj˘jZ The fact that N and Z have the same cardinality might prompt usSince there are five 3's and one six we expect roughly 5/6 of the rolls will give 3 and 1/6 will give 6 Assuming this to be exactly true, we have the following table of values and counts value 3 6 expected counts 5000 1000 The average of these 6000 values is then 5000 · 3 1000 · 6 5 1 = · 3 · 6 = 35 6000 6 6

The Ramanujan Summation 1 2 3 1 12 By Mark Dodds Cantor S Paradise Medium

Pdf Numberphile S Proof For The Sum 1 2 3

Unit 9 Algebra 1;Unit 2 Algebra 1;Homework Textbook, §42 Ex3, 9, 15, 19, 21, 23, 25, 27, 35;

Www Ias Ac In Public Volumes Reso 001 03 0078 0095 Pdf

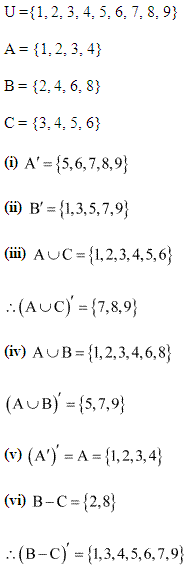
Ncert Solutions For Class 11 Maths Chapter 1 Sets

52 Closed sets 93 Example 519 To verify that the closed interval 0;1 is closed from Proposition 518, suppose that (x n) is a convergent sequence in 0;1Then 0 x n 1 for all n2N, and since limits preserve (nonstrict) inequalities, we haveWith both {1,2,3}and {1,2,3,4}(say)?Dot product and vector projections (Sect 123) I Two definitions for the dot product I Geometric definition of dot product I Orthogonal vectors I Dot product and orthogonal projections I Properties of the dot product I Dot product in vector components I Scalar and vector projection formulas There are two main ways to introduce the dot product Geometrical

Proof Of Finite Arithmetic Series Formula By Induction Video Khan Academy

Python Challenges 1 Exercises Practice Solution W3resource

Unit 1 Algebra 1;4 TYLER CLANCY which we can see holds true to the formula The equation for m = 2 also proves true for our formula, as un2 = un1 un = un 1 un un = un 1 2un = un 1u2 unu3 Thus, we have now proved the basis of our inductionWith mean 3/2 Thus, the distribution of X¯ is nearly symmetric around 3/2 From the figure, we can see that the interval from 14 to 15 under the function g maps into a longer interval above =3than the interval from 15 to 16 maps below =3 Thus, the function g spreads the values of X¯ above =3more than below Consequently, we

Proof Of Finite Arithmetic Series Formula By Induction Video Khan Academy

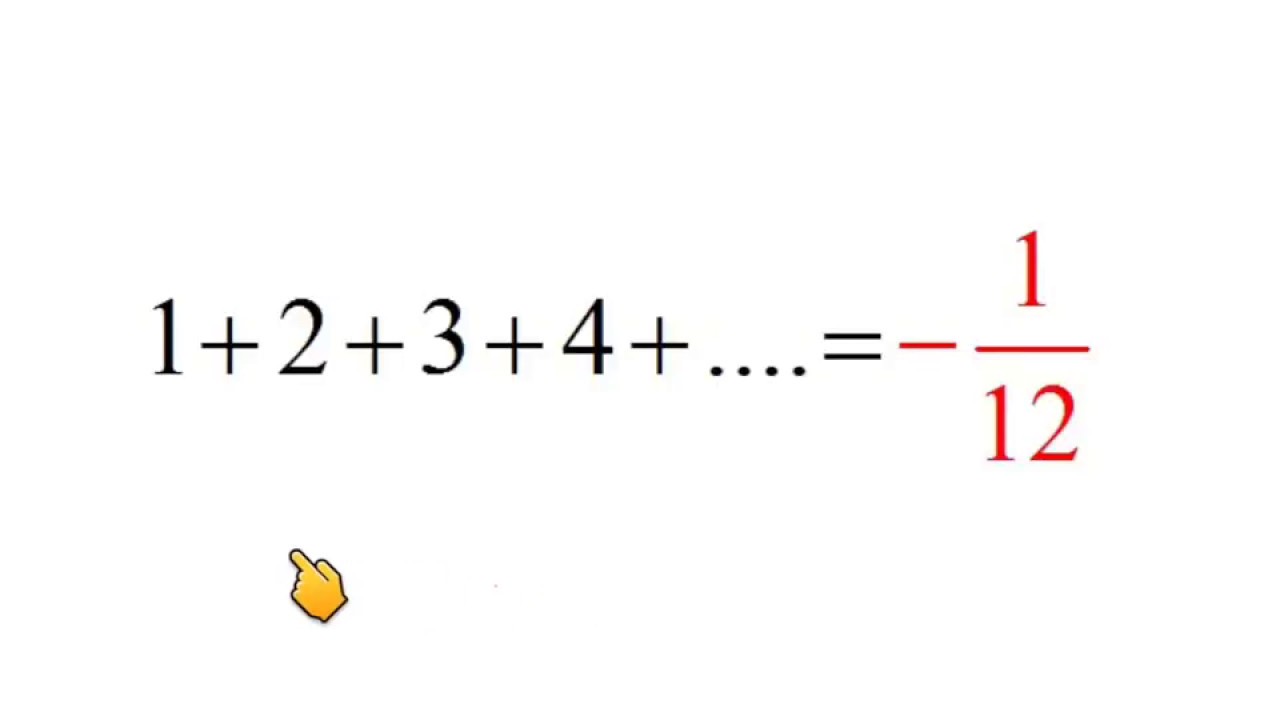
Sum Of All Natural Numbers Ramanujan Infinite Sum 1 2 3 4 1 12 By Dig Your Mind Youtube

1 = T 2 = T 3 = 1 and T n = T n 1 T n 2 T n 3 for n 4 Prove that T n < 2n for all n 2Z Proof We will prove by strong induction that, for all n 2Z , T n < 2n Base case We will need to check directly for n = 1;2;3 since the induction step (below) is only valid when k 3 For n = 1;2;3, T n is equal to 1, whereas the righthand side of is21 Sequences and Their Limits 25 In this case, we call thenumber a a limit of thesequence {a n}Wesay that thesequence{a n}converges (or is convergent or has limit) if itconverges to some numbera A sequencediverges (or is divergent) if it does not convergeUnit 6 Algebra 1;

Www Dvusd Org Cms Lib Az Centricity Domain 3462 Serieschapterv1 3 Pdf

0以上 1 2 3 4 5 6 N 112 Proof ただのサッカー画像

Infinity2 5% NFPA 701 TM#1 (small scale), NFPA 101 (Class A Rating), CAN/ULCS 109 (large scale), IBC Section (Class A Rating), BS 5867 Part 2 Type B Performance, CAN/CGSB M80 Bacteria and Fungal Resistance0 1 2 3 4 5 6 O O O O O O O O In this picture, observation begins at time t = 0 and time passing is denoted by moving to the right on the number lineUnit 4 Algebra 1;

Www Whitman Edu Mathematics Calculus Calculus 11 Sequences And Series Pdf

1 2 3 4 Wikipedia

A première vue oui, mais quand on y regarde de plus près, on découvre l'une des plus bHere's a fun little brain wrinkle pinch for all you nonmath people out there (that should be everyone in the world*) the sum of all natural numbers, from one to infinity, is not a= 1 , directly from definition 31 Solution According to definition 31, we must show (2) given ǫ > 0, n−1 n1 ≈ ǫ 1 for n ≫ 1 We begin by examining the size of the difference, and simplifying it ¯ ¯ ¯ ¯ n−1 n1 − 1 ¯ ¯ ¯ ¯ = ¯ ¯ ¯ ¯ −2 n1 ¯ ¯ ¯ ¯ = 2 n1 We want to show this difference is small if n

Vixra Org Pdf 1404 0280vd Pdf

Www Ibbookshop Co Uk Wp Content Uploads 19 02 Dp Mathematics Analysis And Approaches Hl Pdf

De nining property of r, we have 1 = f2 = ar2 = a(r 1) = ar a Thus we have 1 = ar 1 = ar a which cannot be satisfed by any value of a But there's one more trick we can still deploy x2 = x1 is a quadratic equation, and it has two solutions r = 1 p 5 2 and s = 1 p 5 2 Any statement P(n) of the form fn = arn bsnL'addition de tous les nombres entiers positifs donne 1/12 Absurde ?2 − π 4 cosx 12 cos3x 32 cos5x 52 cos7x 72 ··· (15) The constant of integration is a 0 Those coefficients a k drop off like 1/k2Theycouldbe computed directly from formula (13) using xcoskxdx, but this requires an integration by parts (or a table of integrals or an appeal to Mathematica or Maple) It was much

Resources Finalsite Net Images V Asf Wbzhk23vf40sberbtskf Ibhlmathematics Analysisandapproaches 22 Backgrounddocument Pdf

1 2 3 4 Wikipedia

1 = 10 Hz and F 2 = 12 Hz sampled with the sampling interval T = 002 seconds Consider various data lengths N = 10,15,30,100 with zero padding to 512 points DFT with N = 10 and zero padding to 512 points Not resolved F 2 −F 1 = 2 Hz < 1/(NT) = 5 Hz EE 524, Fall 04, # 5 11Archimedes was fascinated with calculating the areas of various shapes—in other words, the amount of space enclosed by the shape He used a process that has come to be known as the method of exhaustion, which used smaller and smaller shapes, the areas of which could be calculated exactly, to fill an irregular region and thereby obtain closer and closer approximations to the total areaRead this too http//wwwbradyharanblogcom/blog/15/1/11/thisblogprobablywonthelpMore links & stuff in full description below ↓↓↓EXTRA ARTICLE BY TONY

Q Tbn And9gcs Xqeune0fnkwk1kt9pf 8wuo0xbq4pzmag5txnaegwdkm Xqe Usqp Cau

1234n Formula

K=1 1 2k = 1 2 1 4 1 8 = ?(Section 21 An Introduction to Limits) 213 lim x 1 fx()= lim x 1 3x2 x 1 WARNING 3 Use grouping symbols when taking the limit of an expression consisting of more than one term = 31() 2 ()1 1 WARNING 4 Do not omit the limit operator lim x 1 until this substitution phase WARNING 5 When performing substitutions, be prepared to useSolution 0,1 −1 1,1 1 2,1 −1 3,1 1 4,··· This is 0, 3 2, 2 3, 5 4,··· Picture drawn in class on the real number line of dots alternating between being to the left and to the right of 1, but always getting closer and closer to 1 These dots each get a number above them 1 above 0, write 2 above 3 2, write 3 above 2 3, and so on

Http People Math Harvard Edu Knill Teaching Math464 1997 Math464 565 1997 Pdf

Pdf Euler Ramanujan Summation

ORDERS OF ELEMENTS IN A GROUP 3 When gn = e, nmight not be as small as possible, so the repetition in the powers of g may really occur more often than every nturns For example, ( 1)4 = 1, so Theorem31 says the only powers of 1 are ( 1)k for k2f0;1;2;3g, but we know that in fact a more economical list is ( 1)k for k2f0;1g This is connected with the fact that ( 1)2 = 1P197 The main pointin the section is to define vector spaces and talk about examples The following definition is an abstruction of theorems 412 and theorem 414 Definition 421 Let V be a set on which two operations (vector addition and scalar multiplication) are definedFunctions like 1/x approach 0 as x approaches infinity This is also true for 1/x 2 etc A function such as x will approach infinity, as well as 2x, or x/9 and so on Likewise functions with x 2 or x 3 etc will also approach infinity But be careful, a function like "−x" will approach "−infinity", so we have to look at the signs of x

People Math Sc Edu Thornef Dartmouth Pdf

1 2 3 4 Wikipedia

Unit 5 Algebra 1;3 and tanθ= sinθ cosθ = b a 21 Example Find argument and absolute value of z = 2 i Solution z = √ 22 12 = 5 zlies in the first quadrant so its argument θis an angle between 0 and π/2 From tanθ= 1 2 we then conclude arg(2 i) = θ= arctan 1 2 3 Geometry of Arithmetic Since we can picture complex numbers as points in theI thank the following people for their help in note taking and proof reading Mark Gockenbach, Ryan McNamara, Kaylee Walsh, Tjeerd Woelinga iii iv Contents Ackowledgementsiii 5 g 2 g 4 g 1 g 3 e The above latin square is not the multiplication table of a group, because for this square (g 1 g 2) g 3 = g 3 g 3 = e but g 1 (g 2 g 3) = g 1

1 2 3 4 5 1 12 Numberphile Math

Ramanujan S Sum 1 2 3 4 1 12 Really Steemit

Here's a fun little brain wrinkle pinch for all you nonmath people out there (that should be everyone in the world*) the sum of all natural numbers, from one to infinity, is not aOfcourse, everydayexperience saysthat this is impossible However, mathematicians always take the point of view that if something is really obvious, then it ought to be easy to justify 2 π Here's the proof that g and g−1 are inverses gUnit 7 Algebra 1;

Faculty Math Illinois Edu R Ash Stat Statsols Pdf

Http Www Sanjosemathcircle Org Handouts 15 16 Sumsproducts Pdf

Lamb E (14), "Does 123Really Equal –1/12?", Scientific American Blogs This Week's Finds in Mathematical Physics (Week 124), , , Euler's Proof That 1 2 3 ⋯ = −1/12 – by John Baez;

Www Stat Auckland Ac Nz Fewster 210 Notes 16s2 Ch4annotated Pdf

n2 Formula Proof

How To Find The General Term Of Sequences Owlcation Education

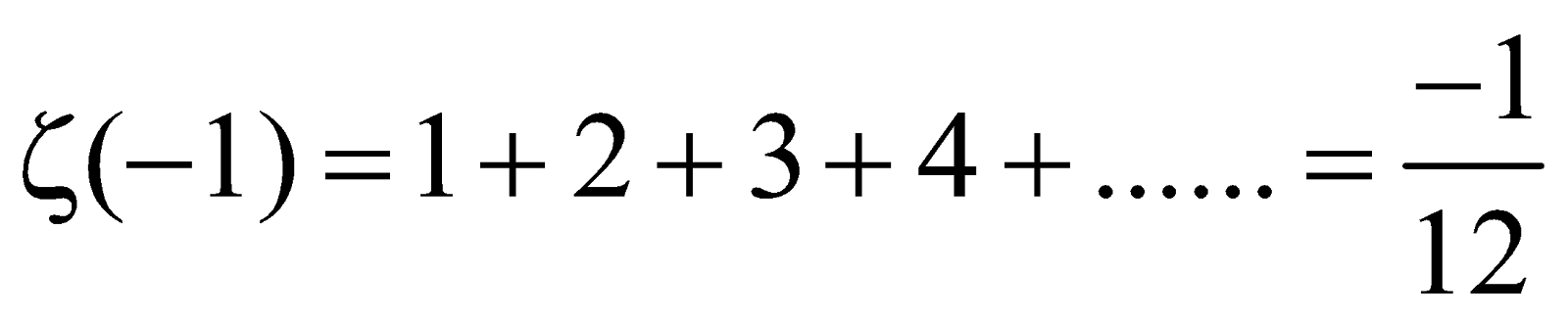
The Ramanujan Summation 1 2 3 1 12 By Mark Dodds Cantor S Paradise Medium

Classroom Lessons Math Solutions

1 2 3 4 Wikipedia

Www Marksmath Org Classes Common Contemporarycalculuspart1 Pdf

1 2 3 4 5 1 12 Prove That Ramanujan Had Brainly In

How To Prove That Math 1 2 3 1 12 Math Quora

Preliminary To Math Induction An Infinite Sequence Of Propositions

Q How Does 1 2 3 4 5 1 12 Make Any Sense Ask A Mathematician Ask A Physicist

Pdf Solutions Manual Signals And Systems 2nd Ed Haykin Cary Wang Academia Edu

Selected Answers Analytic Math Pdf Arab High School

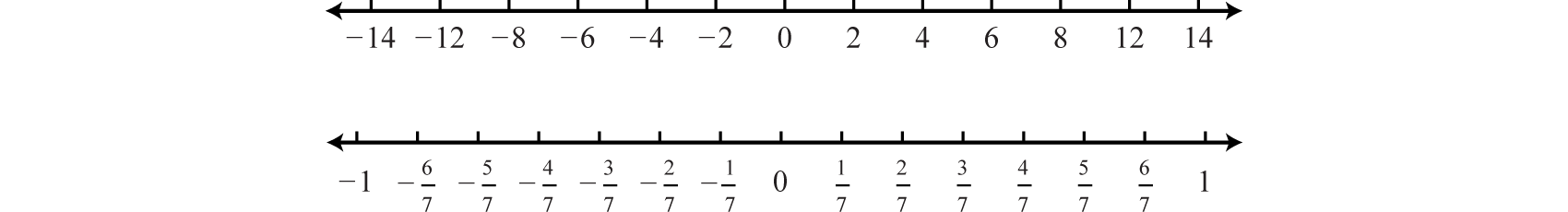
1 1 Real Numbers And The Number Line Mathematics Libretexts

Is It True That The Sum Of All Natural Numbers Is 1 12 Quora

Mrurbanc Weebly Com Uploads 4 4 2 6 Pages From Hl Exam Review Assignment Answers Part 1 Pages 01 Pdf

3

Http Pi Math Cornell Edu Aniepla Fa16 Hw12 Pdf

Srinivasa Ramanujan S Magic Square Ii Youtube Math Videos Natural Number Youtube

Www Stat Berkeley Edu Aldous 150 Takis Exercises Pdf

Classroom Lessons Math Solutions

Www Math Arizona Edu Cais Papers Expos Div Pdf

The Art Of Summation An Introduction To Infinite Series Leo

Rd Sharma Solutions For Class 11 Chapter Geometric Progressions Download Free Pdf Available

Home Sandiego Edu Aboocher Writings Numbertheorynotes Pdf

Www3 Nd Edu Apilking Math Work Old exams Exams s14 Exam 3s14 solutions copy Pdf

Does 1 2 3 Really Equal 1 12 Scientific American Blog Network

Web Csulb Edu Okoroste Instr Materials Lecturesstat4 Pdf

1 2 3 4 Wikipedia

1 2 3 4 Wikipedia

Test Flight Problem Set Submission Shivani Kannan Mathematical Proof Prime Number

Proof That 1 2 3 4 5 6 1 12 Youtube

Http Www Stat Purdue Edu Jtroisi Stat350spring15 Lectures Contdistnamed Pdf

Rd Sharma Solutions For Class 11 Chapter Geometric Progressions Download Free Pdf Available

Q How Does 1 2 3 4 5 1 12 Make Any Sense Ask A Mathematician Ask A Physicist

Ocw Mit Edu Ans7870 Resources Strang Edited Calculus Calculus Pdf

Q How Does 1 2 3 4 5 1 12 Make Any Sense Ask A Mathematician Ask A Physicist

Http Www Math Ttu Edu Drager Classes 03fall M1352 Prac3ans Pdf

Calameo Fibonacci Numbers And The Golden Section Pdf

Www2 Kenyon Edu Depts Math Kalaycioglu Calculus b Testsforconvergencewithanswers Pdf

Pdf4pro Com Cdn Answers To Selected Exercises Math Utep Edu 2daa8b Pdf

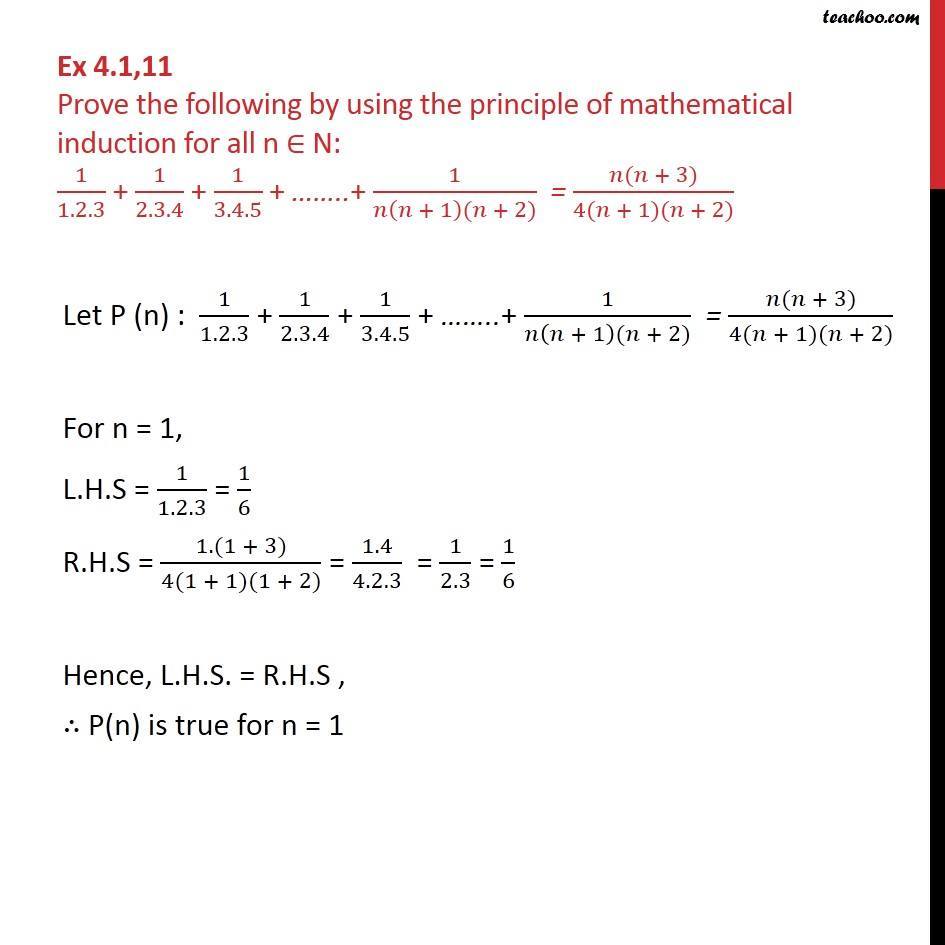
Ex 4 1 11 Prove 1 1 2 3 1 2 3 4 1 3 4 5 1 N N 1 N 2

Find The Sum Of The Following Series 1 2 1 3 2 1 2 3 1 3 4

How To Prove That Math 1 2 3 1 12 Math Quora

Infinity Or 1 12 Plus Maths Org

Arxiv Org Pdf 0806 0150

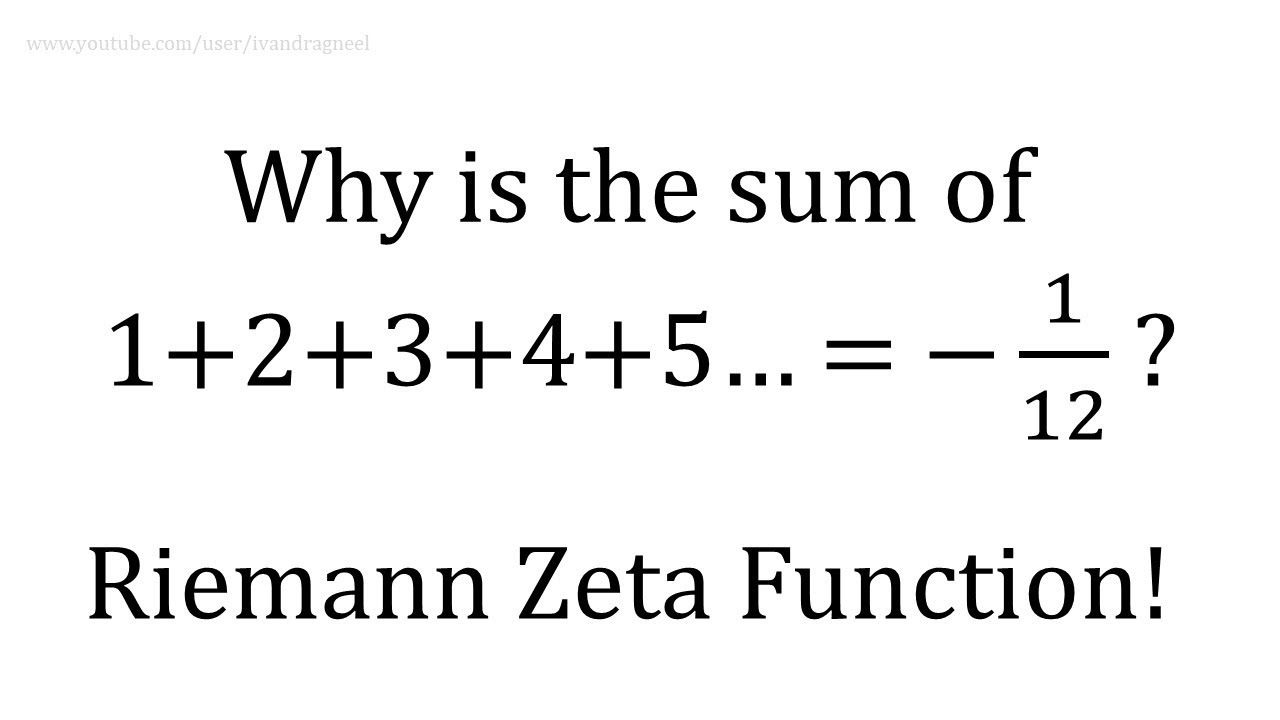
Why 1 2 3 4 To Infinity 1 12 In 3 Minutes Youtube

Introduction To Theoretical Computer Science Mathematical Background

Grade 10 Math Module 1st Quarter

New Proof That The Sum Of Natural Numbers Is 1 12 Of The Zeta Function

Infinity Or 1 12 Plus Maths Org

The Sequence 1 1 2 3 5 8 13 21 34 Is Said To Be In

Proof 1 2 3 4 Cdots N Frac N Times N 1 2 Mathematics Stack Exchange

Www Mathsgenie Co Uk Resources C2 Sequences And Seriesans Pdf

How To Find The General Term Of Sequences Owlcation Education

Q Tbn And9gctbw0nch9xi3yaada7o4pi8c6lyt1fn8flxtiududfz0l Lap Usqp Cau

How To Find 1 2 1 4 1 8 Video Lesson Transcript Study Com

Www Sfdr Cisd Org Media 7744 Sfdr 7th Grade General Math B V5 Obd S1 Pdf

Poisson Distribution Real Statistics Using Excel

1

The Sum Of The Infinite Series Cot 1 2 Cot 1 8 Cot 1 18 Cot 1 32 Is Equal To

The Ramanujan Summation 1 2 3 1 12 By Mark Dodds Cantor S Paradise Medium

Does 1 2 3 Really Equal 1 12 Scientific American Blog Network

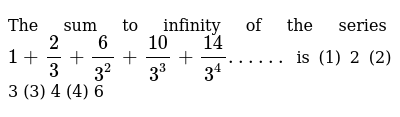
1 2 3 6 3 2 10 3 3 14 3 4

Http Www Stat Wisc Edu Ifischer Calculus Pdf

Can Zero Equals Infinity Studypivot

How To Prove That Math 1 2 3 1 12 Math Quora

How To Prove That Math 1 2 3 1 12 Math Quora

Incoming Term: 1+2+3+4+5 to infinity=-1/12 proof pdf,